Equiangular Segments

Equiangular segments are an interesting occurrence in geometry. We will discover the fascinating segments, their concurrency, and their length. These segments can be external or internal.

External Equiangular Segments

If equilateral triangles are erected externally on the sides of any arbitrary triangle and segments from the vertices of the equilateral triangles that do not touch the arbitrary triangle are drawn to the corresponding vertices of the arbitrary triangle, then the three segments are equal in length and are concurrent.


View angles View angles     View lengths View lengths

In the figure:

  • The length of the external equiangular segments, pe, in terms of the sides of the arbitrary triangle is given by the formula: \({\overline{AA'}=\overline{BB'}=\overline{CC'}=p_{e}}\) and \({p_{e}^2=\frac{1}{2}(a^2+b^2+c^2)+2\sqrt{3}K}\), where a, b, and c are the sides of the arbitrary triangle and K is the area of the arbitrary triangle.
  • The equiangular segments are concurrent at a common point O. Point O is the equiangular point of ΔABC: ∠AOB = ∠BOC = ∠COA = 120°. Moreover, all of the 6 angles at O are equal to 60°.
  • AOCB', BOAC', and COBA' are cyclic quadrilaterals.

Proof of Congruency and Cyclic Quadrilaterals

By congruency, we mean that AA', BB', and CC' are equal in length. This is proved with the SAS congruency criterion. Using SAS criterion, two triangles are congruent if two sides are equal in length and the include angle is equal.

In triangles B'CB and ACA', two of the sides are equal, where B'C = AC and CB = CA'. Also, angle ∠B'CB = 60° + C and angle ∠ACA' = C + 60°. Hence, by SAS, the two triangles are congruent. This means that the third sides, AA' and BB', of each triangle are equal in length. Using the same method, we can prove that the third segment CC' is also equal to the other two.

(i) AA' = BB' = CC'

To prove that the quadrilaterals AOCB', BOAC', and COBA' are cyclic, we notice that ∠CA'A = ∠CBB' because the triangles are congruent. Its measure is denoted by α1. Similarly ∠C'CB = ∠AA'B = α2. Also, α1 + α2 = 60°. The sum of the internal angles of a quadrilateral = 360°. We can use simple math to conclude that ∠COB = 120°. Since ∠COB + ∠CA'B = 120° + 60° = 180°, the quadrilateral COBA' must be cyclic because the opposite angles in a cyclic quadrilateral equal 180°. We can make the same conclusions for the other two quadrilaterals.

(ii) AOCB', BOAC', and COBA' are cyclic quadrilaterals

Proof of Concurrency

After having proved the aforementioned quadrilaterals are cyclic, we can easily prove the concurrency of the three equiangular segments with Ceva's Theorem. Since AOCB' is a cyclic quadrilateral, we have \(\frac{v}{r} = \frac{\overline{AB'}}{l} = \frac{b}{l}\) and \({\frac{v}{z}=\frac{\overline{CB'}}{j}=\frac{b}{j}}\). Therefore, \({\frac{z}{r}=\frac{j}{l}}\). This property is a property of angle bisectors. Therefore, OB' must be the angle bisector of ∠AOC. Since ∠AOC = 120°, ∠COB' = ∠AOB' = 60°. Similarly, all of the equiangular segments are angle bisectors. To summarize: \({\frac{x}{p}=\frac{l}{k}}\), \({\frac{y}{q}=\frac{k}{j}}\), and \({\frac{z}{r}=\frac{j}{l}}\). Thus, by Ceva’s Theorem, the segments are concurrent since \({\frac{x}{p}\cdot\frac{y}{q}\cdot \frac{z}{r}=\frac{l}{k}\cdot\frac{k}{j}\cdot\frac{j}{l}=1}\).

Derivation of the Length Formula

Next problem we tackle is to determine the length of the equiangular segment. Because the segments are known to be equal in length, we can use the Law of Cosines to state the following:

(i) \({p_{e}^2=b^2+c^2-2bc\cdot\cos(60+A)}\)

(ii) \({p_{e}^2=b^2+c^2-}\) \({2bc(\cos{60}\cdot\cos{A}-\sin{60}\cdot\sin{A})}\)

The area of a triangle, K, is given by the formula: \(K = \frac{1}{2}bc\sin A\). We can substitute that into equation (ii). Also, \(\cos A\) can be found using the Law of Cosines.

(iii) \(p_{e}^2=b^2+c^2-\) \(2bc\left(\frac{1}{2}\cdot\frac{b^2+c^2-a^2}{2bc}-\frac{\sqrt{3}}{2}\cdot\frac{2K}{bc}\right)\)  (Expanding and collecting using the sum property of Cosine)

(iv) \({p_{e}^2=\frac{a^2+b^2+c^2}{2}+2\sqrt{3}K}\)   where K is the area of the triangle

Internal Equiangular Segments

The properties of the internal equiangular segments are similar to the properties of the external equiangular segments as noted below.

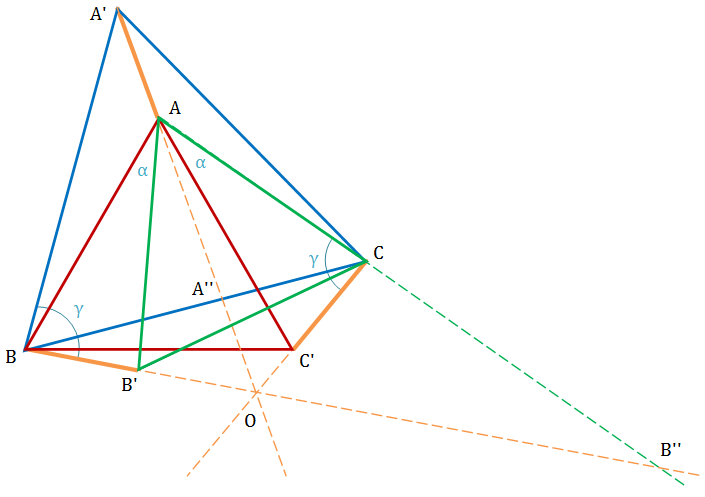Internal equiangular segmentsIn the figure:
  • The lengths of the internal equiangular segments, AA', BB', and CC', are equal in length.
  • The segments, if extended, are concurrent at O.
  • The six angles created by point O are all equal to 60°.
  • Quadrilaterals ABOC', OCAB', and BOCA' are cyclic. All of these cyclic quadrilaterals have the common angle ∠BOC = 120°.
  • The proof of concurrency is similar to the proof of concurrency for the external counterpart. The only difference is that point O lies outside the triangle.
  • Point C'' is not shown since it lies well beyond this page!
  • Angles α and γ are shown to prove that ΔACC' ≅ ΔAB'B by SAS criterion. Thus, CC' = BB'.

If the equilateral triangles are created on the inside of the triangle ABC, then the internal equiangular segments, AA', BB', and CC' are again equal in length. The length of the segments, pi, is given by \({\overline{AA'}=\overline{BB'}=\overline{CC'}=p_{i}}\) and \({p_{i}^2=\frac{1}{2}(a^2+b^2+c^2)-2\sqrt{3}K}\). By simple addition, we also have \({p_{e}^2+p_{i}^2=a^2+b^2+c^2}\).

Proof of Concurrency

To prove that the internal equiangular segments are concurrent, we need to prove that \({\left(\frac{\overline{AB''}}{\overline{-B''C}}\right)\left(\frac{\overline{CA''}}{\overline{A''B}}\right)\left(\frac{\overline{BC''}}{\overline{-C''A}}\right)=1}\). The negative sign indicates a point lying on the line continuous with the segment on the side of the triangle rather than on the side of the triangle itself. For example, point B'' does not lie on the side of the triangle but on a line continuous with the side.

In triangle AOB'', we know that OC is the angle bisector of ∠AOB''. Therefore:

(i) \({ \frac{AB''}{-B''C}= -\frac{OA}{OC} }\).

Similarly, from triangle BOC, we have:

(ii) \({ \frac{AB''}{B''C}= \frac{OC}{OB} }\).

Since C'' lies beyond this page, triangle AOC'' is not shown. The important relationship we obtain from this triangle is:

(iii) \({ \frac{BC'}{-C''A}= -\frac{OB}{OA} }\).

Multiplying these relationships, we obtain 1; thus, completing the proof of concurrency.